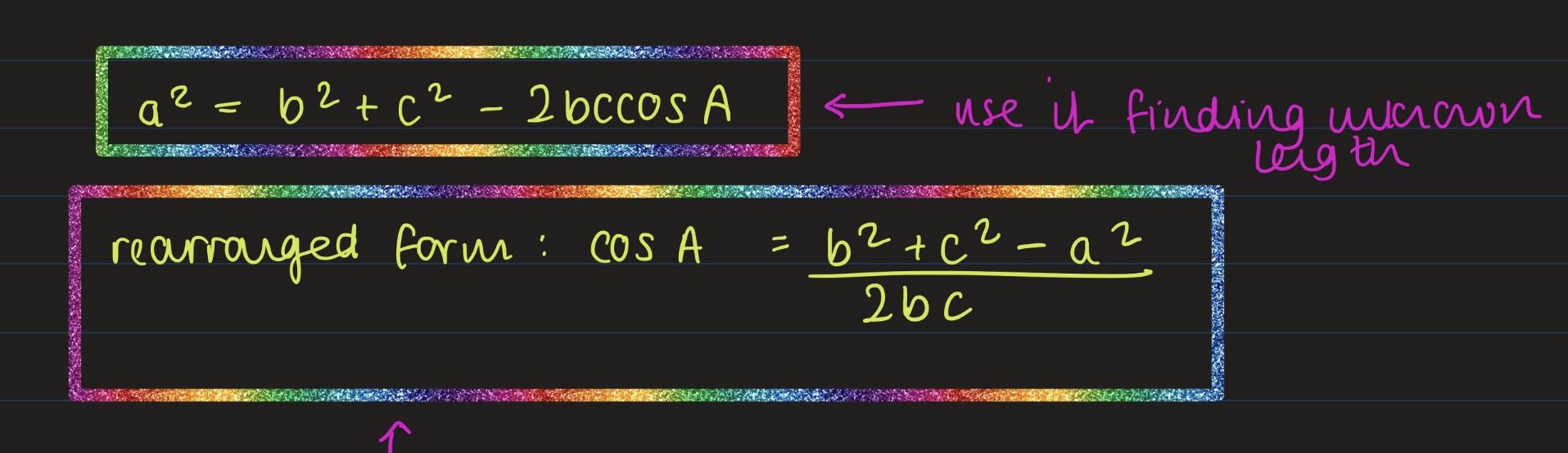Algebra and Functions
The Modulus Function
- Understand and Use the Modulus Function
- Solve Equations and Inequalities involving the Modulus
- Solve Equations and Inequalities involving the Modulus Graphically
- Sketch the Modulus and the Modulus of Linear Functions
Functions
- Understand and be able to use the definiton of a function
- Vocabulary and associated notation i.e. many-one, one-many, one-one, mapping, image, range, domain
- Definitions of range and domain
- Understand and be able to use inverse functions and their graphs
- Know the conditions for the inverse function to exist
- Be able to find the inverse of a function either graphically, by reflection in the line y = x, or algebraically
- Composite functions
Graph Transformations
Coordinate Geometry
Circles
- Understand and be able to use the coordinate geometry of a circle including using the equation of a circle
- Be able to draw a circle given its euation or form the equation given its centre and radius
- Be able to complete the square to find the centre and radius of a circle
- Be able to investigate wheter a line and a circle or two circles intersect
Trigonometry
Radians
- Be able to work with radian measure
- Use the relationship between degrees and radians
- Know and able to use exact values of sin x, cos x, tan x (table in notes)
- Extend knowledge of trigonmetric equations and identities to include radians
- Be able to work with radian measure and use for arc length and sector
Small Angle Approximations
- Understand and be able to use the standard small angle approximations
Compound & Double Angle Formulae
- Understand and be able to use the double angle formulae and compound angle formulae
- Understand the geometric proofs of these formulae
- Be able to prove the double angle formulae
- Be able to construct proofs involving trigonometric functions and identities
- Use the formulae to solve trigonometric equations, prove identities or evaluate integrals
Exponentials and Logarithms
Introduction
- Know and use the function ax and its graph, where a is positive
- Know and use the function ex and its graph (examples may include the comparison of two population models or models in a financial or biological context and the link with geometric sequences may be made).
- Know the gradient of ekx is equal to kekx and hence understand why the exponential model is suitable in many applications.
- Know and use the definition of logax (for x > 0) as the inverse of ax (for all x), where a is positive.
- Be able to convert from index to logarithmic form and vice versa.
- Know and use the function ln x and its graph.
- Know and use ln x as the inverse of ex.
Laws of Logarithms
- Understand and be able to use the laws of logarithms.
Exponential Equations
- Be able to solve equations of the form ax = b for a > 0.
- Solve equations which can be reduced to this form such as 2x = 32x - 1, either by reduction or by taking logarithms of both sides.
Modelling
- Be able to use logarithmic graphs to estimate parameters in relationships of the form y = axn and y = kbx, given data for x and y.
- Be able to reduce equations of these forms to a linear form and hence estimate values of a and n, or k and b by drawing graphs using given experimental data and using appropriate calculator functions.
- Understand and be able to use exponential growth and decay and use the exponential function in modelling.
- Be able to use of e in continuous compound interest, radioactive decay, drug concentration decay and exponential growth as a model for population growth.
- Consider limitations and refinements of exponential models.
Differentiation
Differentiation of Trigonometric Functions
- Be able to show differentiation from first principles for sin x and cos x.
- Be able to differentiate sin kx, cos kx, tan kx and related sums, differences and constant multiples.
Differentiation of Exponentials and Logarithms
- Be able to differentiate ekx and akx, and related sums, differences and multiples.
- Understand and be able to use the derivative of ln x.
The Chain Rule
- Be able to differentiate using the chain rule.
The Product Rule
- Be able to differentiate using the product rule and quotient rule.
The Quotient Rule
- Be able to differentiate using the product rule and quotient rule.
Implicit Differentiation
- Be able to differentiate simple functiosn and relations defined implicitly (for the first derivative only).
Statistical Sampling
- Understand and be able to use the terms population and sample.
- Be able to use samples to make informal inferences about the population.
- Understand and be able to use sampling techniques including simple random sampling and opportunity sampling.
- Select or critique sampling technqiues in the context of solving a statistical problem.
- Understand that different samples can lead to different conclusions about the population.
- Be familiar with and be able to critique systematic, stratified, cluster and quota sampling.
Data Presentation and Interpretation
- Interpret tables and diagrams for single-variable data: vertical line charts, dot plots, bar charts, stem-and-leaf diagrams, box-and-whisker plots, cumulative frequency diagrams and histograms.
- Understand, in context, the advantages and disadvantages of different statistical diagrams.
- Interpret scatter diagrams and regression lines for bivariate data.
- Recognise scatter diagram which include distinct sections of the population.
- Understand informal interpretation of correlation.
- Understand that correlation does not imply causation.
- Calculate and interpret measures of central tendency and variation including mean,median,mode,percentile,quartile,inter-quartile range, standard deviation and variance.
- Understand standard deviation is the root mean square deviation from the mean.
- Use the mean and standard deviation to compare distributions.
- Calculate mean and standard deviation from a list of data, from summary statistics or a frequency distribution using calculator statistical functions.
- Understand in the case of grouped frequency distribution the calculated mean and standard deviation are estimates.
- Recognise and be able to interpet possible outliers in data sets and statistical diagrams.
- Select or critique data presentation techniques in the context of a statistical problem.
- Be able to clean data, including dealing with missing data, errors and outliers.